In our last article, we explained the forward pass step in the critical path method. We showed the official approach, where the project starts at day 1, and an approach that is simpler to calculate in a PMP© exam situation, where the project starts at day 0. In considering the backward pass, we will use the same example and again offer the 1-based and 0-based solutions.

When we had worked through the forward pass using the 1-based technique, our network diagram was:

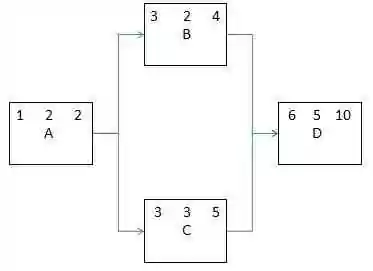
In order to identify the critical path and to detect float in the other paths, we need to carry out a backward pass. This augments the diagram as follows:

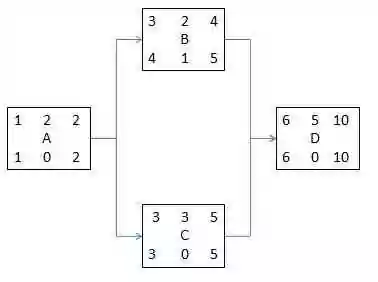
Just like the forward pass, the backward pass requires some tricky calculations. We begin, by taking the largest Early Finish in the diagram (that of activity D in this case) and subtracting the estimated duration. This gives us the Late Finish for the preceding activities (B and C).To get the Late Start for activity D, we need to add one to this figure. Now, the float (0 in the case of activity D) is calculated by subtracting the Early Start from the Late Start.

With activity B, we do the same series of operations. Subtract the duration (2 days) from the Late Finish (day 5). Because our predecessor activity (activity A) has two successors, we will have to determine which of these will have the smaller value. This will become the Late Finish for activity A. If activity B has the smaller value, the Late Finish of activity A will be 3. To get the Late Start for activity B, we need to add one to this figure to obtain 4. Subtracting the Early Start from the Late Start gives us 1 day’s worth of float.
Activity C is calculated the same way. Its suggestion for activity A’s Late Finish is activity C’s Late Finish (5) – its estimated duration (3) = 2. This is smaller than what activity B proposes (3), so we will use this value instead. To complete the values for activity C, we need to add 1 to activity A’s Late Finish to obtain the Late Start (3) and then subtract the Early Start from the Late Start to get the float – which is 0 here.
Activity A now has a Late Finish of 2 (the smaller of its successor’s Late Starts – 1). So subtract activity A’s estimated duration (2) and add 1 to get its Late Start (1). Subtracting the Early Start from the Late Start gives a float of 0. In other words, the critical path is ACD.

Using Velopi’s 0-based technique, we need to start with a different forward pass:

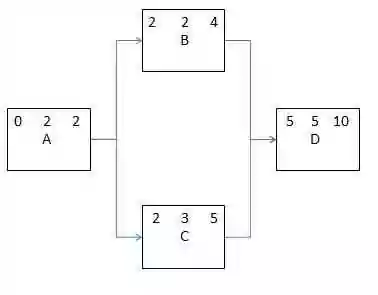
Now, the procedure is to take the Early Finish for the last activity (activity D) and make it the Late Finish:

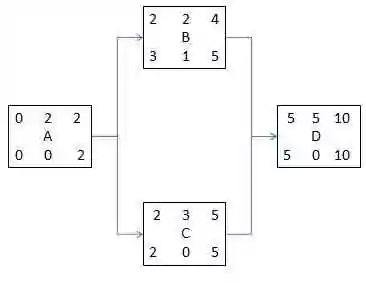
Now subtract activity D’s estimated duration (5) to give a Late Start of 5. Subtract activity D’s Early Start (5) from its Late Start (5) to obtain a float of 0.

Moving backwards, both activities B and C are assigned activity D’s Late Start value (5) as their Late Finishes. Subtracting their estimated durations gives an Early Start of 3 for activity B and an Early Start of 2 for activity C. Subtracting the Early Starts from the Late Starts gives us the float. This is 1 for activity B and 0 for activity C.

Finally, activity A’s Late Finish is the lowest Late Start of its successors. In this case, it is the lower of activity B’s Late Start (3) and activity C’s Late Start (2). Thus activity A’s Late Finish becomes 2. Its estimated duration (2) is subtracted from this Late Finish (2) to give us day 0 as the Late Start. Subtracting activity A’s Early Start from its Late Start gives a float of 0 and the critical path is once more ACD.

Again, it is straightforward to convert this into 1-based values: Simply add 1 to each Late Start.
We have found the 0-based technique for calculating the forward and backward passes very effective from a PMP© exam perspective. It is much easier to calculate the various start and finish dates and the finished product can be converted to 1-based by simply incrementing each start day by 1.